When dealing with optical surfaces, understanding the relationship between different types of surface errors—peak-to-valley (PV), root mean square (RMS), and slope error—is crucial. Here’s how to convert between these error metrics:

- PV (Peak-to-Valley) Surface Error:
- PV surface error is the maximum deviation between the highest and lowest points on the surface.
- RMS (Root Mean Square) Surface Error:
- RMS surface error is a statistical measure of the surface deviations and provides a more comprehensive description of the overall surface quality.
- Slope Error:
- Slope error refers to the angular deviation of the surface normals from the ideal shape, usually given in microradians (µrad) or arcseconds.
Conversion between PV and RMS Surface Error
There is no exact conversion factor between PV and RMS optical surface errors since PV measures the extreme points while RMS measures the overall deviation. However, for most optical surfaces, empirical relationships are often used:
- A common rule of thumb is that RMS error is approximately 1/4 to 1/5 of the PV error for typical surfaces.
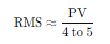
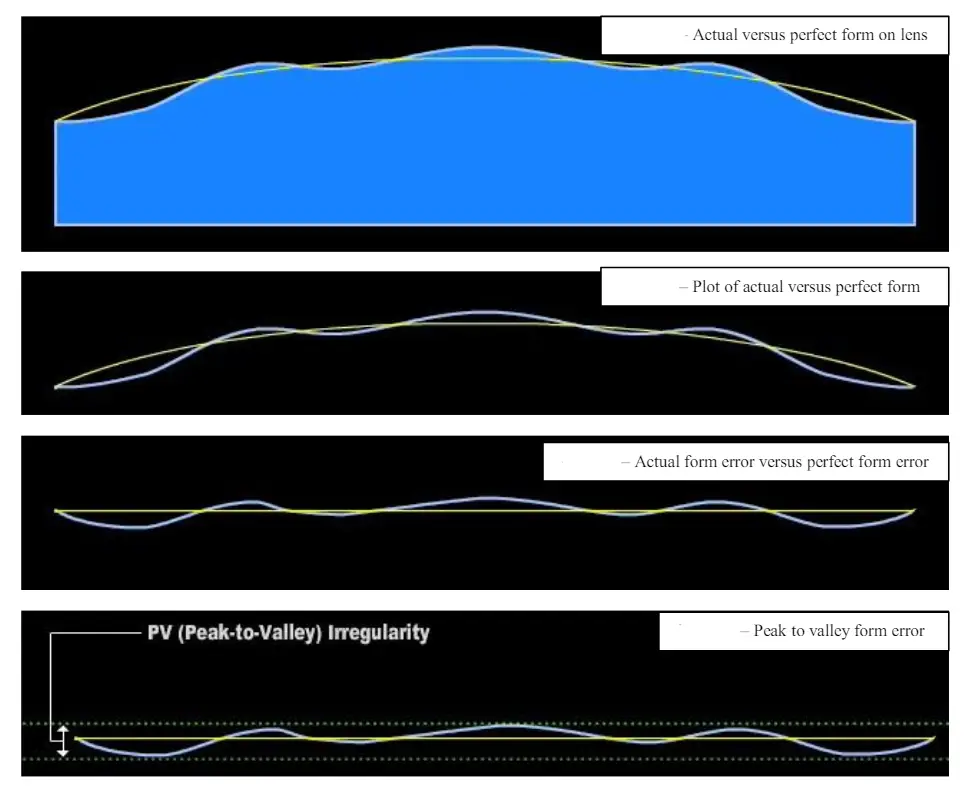
Conversion between Surface Error and Slope Error
Slope error can be related to surface error by considering the derivative of the surface profile. For small slope errors, the relationship can be approximated as:
- Slope Error (in radians) ≈ Surface Error / Spatial Frequency
- For an optical surface with height deviations over a certain spatial frequency, the slope error can be approximated by:

Example Calculation
- Convert PV to RMS: Suppose the PV surface error is 100 nm.RMS≈100 nm4=25 nm
- Convert Surface Error to Slope Error:
Summary
- PV to RMS: RMS ≈ PV / 4 to 5
- Surface Error to Slope Error: Slope Error (µrad) ≈ (Surface Error (nm) / Spatial Frequency (mm)) × 1000
How to measure the Optical Surface Errors above, please contact Shape Optics technical engineer for more information.



